A bell that sounds two notes at once

Have you ever wondered why when you strike a bell (or in this case, a bowl) the sound usually oscillates between louder and quieter while it rings out instead of just slowly becoming quieter? This is what this article is about.

This phenomenon is easier to illustrate with a pendulum that can swing in two directions. the string is tied into a 'Y' at the top, with both ends clamped to the bar above. If I swing the pendulum perpendicular to the bar, the length of the pendulum is from the weight to where the strings are attached to the bar. But if I swing it parallel to the bar, then the effective length is from the weight to the knot that makes a 'Y', a shorter length. Because the pendulum's period of oscillation is in proportion to its length, the pendulum swings faster on the axis parallel to the bar than perpendicular to it.

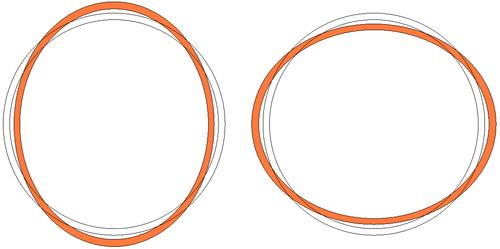
If I swing the pendulum diagonal to the bar, rather than tracing out a straight line, the path of the weight forms a Lissajous curve. But viewed horizontally, at an angle to the bar, it looks like the pendulum's swing increases and decreases with time. What you actually see is the sum of two sinusoidal swings of different frequencies. When they are in phase, the swing looks strong, but when they are out of phase, the only swing is towards / away from the viewer, and the swing doesn't look big.

Words don't really do this justice - I'd recommend you watch the video at the top of this article.

The bell's sound alternating between louder and weaker is also the result of two sine waves of slightly different frequencies which sometimes times add up and at other times oppose, partially cancelling each other.

In terms of how a bell rings at two frequencies, you can think of a bell as a cylinder. The main mode of vibration is that the cylinder alternately stretches vertically (while compressing horizontally), and swings back to stretching horizontally while compressing vertically.

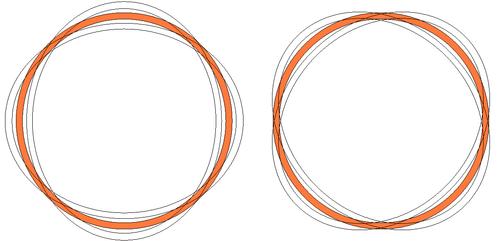But this mode of vibration (left image at left) is independent of vibrations at a 45-degree angle to that (right image at left). Due to imperfections, either deliberate or accidental, these two modes of vibration are often at slightly different frequencies.

I figured it would be a fun experiment to modify it to move the two frequencies of oscillation further apart.

I ground two flat spots on opposite sides of the pipe. Already, this made it sound quite different, making two notes about a semitone (6% change in pitch) apart.

I ground it some more, grinding four flat spots on it corresponding to the nodes of one mode of vibration or the peaks of the other main mode of vibration.

Striking the cylinder half way between the flat spots excites only the higher frequency mode of vibration. I measured it at 1100 Hz, or just below C-sharp two octaves above middle C on a piano. The guitar tuner is able to pick up the note.

The other mode of vibration is 864 Hz, or about a third of a semitone below the second A above middle C. The ratio between these is about 1.27, an interval slightly larger than 5/4 or a "major third" in terms of music.

Playing the two notes the bell plays on the piano while the bell is ringing. The sound is very similar.

I should add that I wasn't trying to tune to any particular musical note or interval with my "bell", it just happened to work out that way. It's difficult to tune such a bell to hit two specific notes because any grinding done to it will affect both notes.

But the coolest feature of this "bell" is that if I strike it so both notes sound, then spin it, you can hear the two pitches alternate. That's because each of the two main modes of vibration sends sound in different directions.